Function

f(x,y) = px+qy

Domain

x and y are elements of the set of all Real numbers

Graphs

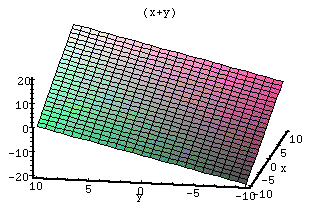
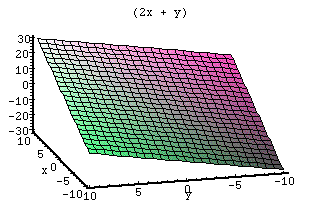
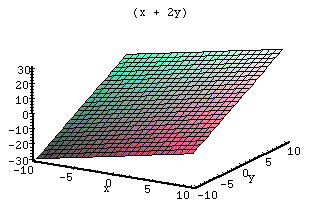
Derivative

With respect to x: p
With respect to y: q

Critical points are formed where the two derivatives equal 0. These critical points may be minimums, maximums, or saddle points. In the first graph, for x, p = 1 and will never equal 0. Therefore, there will be no critical points for this graph. This is shown since there is no saddle point and the curve goes from negative to positive infinity.

Integral

With respect to x: ( ¹/₂ )px² + qxy
With respect to y: pxy + ( ¹/₂ )qy²

Interesting Features

This function is a plane that goes up on an angle right in the middle of the axis. The plane is "flat" and has a constant slope. The value of p causes the table to tilt one way while the q values tilts it in the opposite direction.